Are you ready for a brain-teasing challenge that will twist your mind and put your problem-solving skills to the test? Today’s puzzle might look straightforward, but don’t be fooled by its simplicity. This classic grid puzzle is a perfect way to boost critical thinking and recognize patterns that are not always obvious at first glance. Let’s dive in!
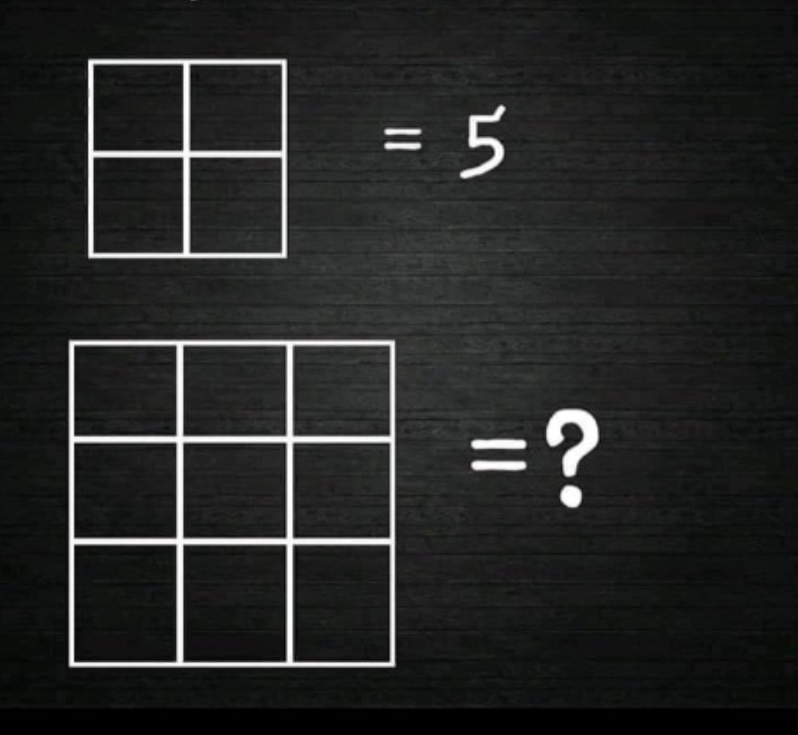
The puzzle begins with an image of a small grid equating to the number 5. The question is: what number does a larger grid represent? If you haven’t yet attempted the puzzle, take a moment to figure it out before reading on—sometimes, the simplest solutions can be the most elusive!
Common Mistakes Solvers Make
When attempting puzzles like this, many people fall into predictable traps. Let’s review a few common errors to avoid:
Miscounting Elements: One of the biggest challenges in puzzles involving grids is failing to account for all elements. People often count the most visible squares but miss some hidden components. To solve the puzzle accurately, it’s essential to think beyond the obvious.
Overcomplicating the Solution: Another frequent mistake is overthinking. Solvers may assume that complex arithmetic or advanced strategies are required when, in reality, a simpler, straightforward approach often works best. The trick is to avoid overcomplicating things and stick with basic logical steps.
Now that we’ve discussed some pitfalls, let’s break down the solution step by step.
Step 1: Understanding the Basic Unit
The first clue in this puzzle shows a 2×2 grid and claims that it equals 5. To understand why, let’s analyze this closely. You might be tempted to think of the grid as just four individual squares, but there’s more going on here. The key is recognizing that in addition to those small squares, the grid itself forms a larger square.
- Small squares: There are four individual 1×1 squares in the 2×2 grid.
- Larger square: The entire grid forms one larger square as well.
Thus, the calculation becomes:
4 small squares + 1 large square = 5 squares total.
Step 2: Applying the Logic to a Larger Grid
Now that we’ve cracked the code for the 2×2 grid, let’s apply the same thinking to a larger 3×3 grid.
- Small squares: A 3×3 grid has nine small 1×1 squares.
- 2×2 squares: In a 3×3 grid, you can also group smaller squares into 2×2 units. How many can you form? The answer is four.
- Large square: Finally, the entire grid forms one large 3×3 square.
So, we count:
9 small squares + 4 2×2 squares + 1 large square = 14 squares in total.
Step 3: Recognizing the Pattern
By now, you should see the emerging pattern: the larger the grid, the more layers of squares you can identify. First, you count the smallest squares. Then, you count larger squares formed by grouping smaller squares together, and finally, you add the largest square representing the entire grid.
This method of counting squares can be applied to puzzles of various grid sizes. In larger grids, additional patterns and square groupings will emerge, but the underlying logic remains the same. It’s all about spotting hidden layers within the grid.
Enhancing Critical Thinking Skills
Why are puzzles like this so effective at building critical thinking skills? Because they encourage you to:
- Think systematically: By breaking the grid down into smaller parts, you ensure that no component is overlooked.
- Apply patterns: Once you’ve identified how the pattern works for a smaller grid, you can use that same logic to solve larger, more complex grids.
- Practice attention to detail: Simple though it may seem, counting squares requires careful observation and an eye for detail.
Puzzles like these can help in real-world applications too. For instance, software developers often need to break problems down into smaller parts and spot patterns in code. Similarly, architects and designers use grids to visualize space and structure.
Step 4: Test Yourself on Other Puzzles
Now that you’ve solved this grid puzzle, why not challenge yourself with other similar puzzles? The logic of counting and pattern recognition can be applied to a wide range of problems, from Sudoku to geometric brain teasers.
Conclusion: Patterns Are Everywhere
The beauty of this grid puzzle is that it teaches us to see patterns that are often hidden in plain sight. By methodically counting all the squares, including those that might not be immediately obvious, we can arrive at the correct solution. The grid puzzle isn’t just about numbers—it’s about seeing the whole picture.
So, the next time you’re faced with a seemingly simple puzzle, remember to pause and think carefully. Sometimes the answer is right in front of you, waiting to be uncovered.
What did you think of this puzzle? Have you come across similar brain teasers that challenged your thinking in a unique way? Feel free to comment below with your thoughts or share any interesting puzzles you’ve encountered. Let’s keep the conversation going and continue challenging our brains together!