Math puzzles may appear simple at first glance, but they often challenge even the sharpest minds. One such classic example is the expression 2 + 6 ÷ 2 × 3. At first, it seems straightforward, but as you begin to solve it, different approaches can lead to varying answers. This puzzle not only tests your math skills but also strengthens your logical thinking. Let’s dive into why this happens and how understanding the rules of math can make solving such puzzles both fun and rewarding.
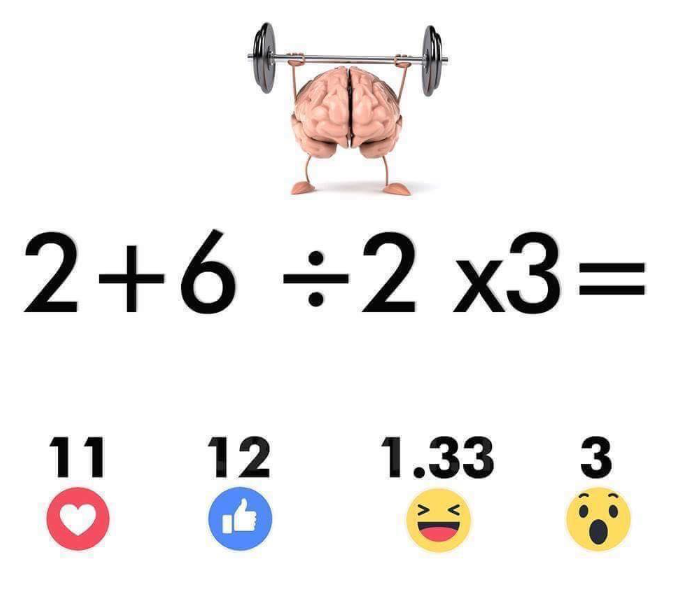
Why Do Simple Math Expressions Create So Much Confusion?
If you’ve ever argued with a friend or scrolled through heated debates online about math puzzles, you’re not alone. Problems like 2 + 6 ÷ 2 × 3 often divide opinions because of misunderstandings or forgotten math rules.
The confusion typically boils down to two factors:
- Misinterpreting the Order of Operations: People often overlook or misapply the established rules for solving math expressions.
- Assumptions About Prioritization: Some mistakenly believe multiplication always comes before division, or addition always follows multiplication, regardless of their order in the expression.
To solve these puzzles accurately, you need to follow a structured approach based on established rules.
The Order of Operations: Your Key to Solving Any Math Problem
The order of operations dictates the sequence in which you solve parts of a math expression. It’s commonly remembered using the acronym PEMDAS:
- P: Parentheses
- E: Exponents
- M/D: Multiplication and Division (from left to right)
- A/S: Addition and Subtraction (from left to right)
One key rule is that multiplication and division are of equal priority, so you solve them in the order they appear, left to right. The same applies to addition and subtraction. Misinterpreting or skipping these rules can lead to incorrect answers.
Breaking Down the Puzzle: 2 + 6 ÷ 2 × 3
Let’s solve the expression step by step, following PEMDAS and paying close attention to the left-to-right rule.
Step 1: Identify the Operations
The expression includes addition, division, and multiplication. Based on PEMDAS, you’ll handle the division and multiplication first, then move to addition.
Step 2: Solve Division and Multiplication (Left to Right)
Start with the division:
6 ÷ 2 = 3
The expression now becomes:
2 + 3 × 3
Next, solve the multiplication:
3 × 3 = 9
The expression now simplifies to:
2 + 9
Step 3: Complete the Addition
Finally, add:
2 + 9 = 11
The Final Answer: 11
Common Errors and Why They Happen
If you didn’t get 11, don’t worry—it’s a common mistake! Here are some reasons why people often arrive at different answers:
- Misinterpreting Multiplication and Division Priority
Some assume multiplication always comes before division, leading to an incorrect grouping, such as solving 6 ÷ (2 × 3) instead of left to right. This results in:
6 ÷ 6 = 1 - Ignoring Left-to-Right Rules
Others mistakenly complete all division first, then all multiplication, disrupting the correct sequence. - Overlooking Parentheses-Like Behavior
While the expression lacks parentheses, some people instinctively group operations incorrectly based on mental shortcuts.
These errors highlight the importance of carefully applying PEMDAS and sticking to the rules.
How Math Puzzles Like This Build Logical Thinking
Puzzles like 2 + 6 ÷ 2 × 3 aren’t just about getting the right answer—they’re about training your brain to think critically. Here’s how they help:
- Detail Orientation: You learn to focus on every part of the problem, ensuring no steps are skipped.
- Systematic Problem-Solving: Following logical rules step by step improves your ability to tackle complex challenges.
- Critical Thinking: Math puzzles push you to question assumptions and verify your reasoning.
These skills aren’t limited to math; they’re useful in decision-making, coding, and even everyday problem-solving.
Why You Should Embrace the Challenge
Math puzzles might seem intimidating, but they’re an excellent way to keep your mind sharp. Whether you’re a student brushing up on fundamentals or someone who simply loves a good challenge, puzzles like this offer a rewarding mental workout.
Don’t shy away from mistakes—use them as learning opportunities. Each misstep helps you understand the rules better, making you more confident in tackling similar problems in the future.
Join the Conversation: What’s Your Answer?
Now that you know the correct answer, what was your initial result? Did you follow the rules of PEMDAS, or did you fall into one of the common pitfalls? Share your thought process with friends or colleagues—it’s a great way to learn and spark lively discussions.
Conclusion: Strengthen Your Logical Thinking, One Puzzle at a Time
The expression 2 + 6 ÷ 2 × 3 may look simple, but it’s a classic example of how easy it is to misinterpret math rules. By understanding and applying PEMDAS correctly, you can arrive at the right answer: 11.
More importantly, puzzles like this are a fantastic way to develop logical thinking, attention to detail, and problem-solving skills. The next time you encounter a math challenge, remember to take it step by step, trust the process, and enjoy the journey of solving it.
Ready for more? Keep tackling math puzzles and logic problems—they’re not just brain teasers; they’re tools for lifelong learning and mental fitness.